1. Introduction
Austrian
capital theory (Mises 1912, 1949; Hayek 1931; subsequently developed by Hayek
1933, 1939, 1941) is used to construct and interpret a vector error-correction
model estimated with
Whenever credit is tightened, interest rates rise, and
higher rates of return in production are necessary to compete with financial
instruments, such as relatively higher-yielding government bonds. This is manifested in a shifting of resources
away from early stages of production to later stages, and can be shown as a
shortening of the base of the Hayekian triangle (Figure 1) (Hayek
1931:39). This paper explicitly tests
the main assertion of Austrian business cycle theory, that increasing available
credit beyond actual saving lowers, rather than increases real output and real
investment expenditure.
The
rest of the paper is organized as follows.
The theoretical basis for the paper is briefly developed in section
2. Section 3 discusses some applications
of Austrian business cycle theory in the economics literature. Data sources are documented in section
4. Section 5 develops the methodological
approach applied in section 6. Section 6
presents and interprets the empirical work, consisting of an error-correction
model of real output as measured by real consumption expenditures. This section
presents tests for cointegration followed by estimates of the error-correction
model. Concluding comments are presented
in section 7.
<<Figure
1 about here.>>
2. The Austrian Theory of the Business Cycle
Consider the problem faced
by a capitalist with idle savings to invest, who engages in a specific
productive activity. One use of these
idle savings is to purchase intermediate inputs or goods-in-process, adding
complementary resources such as labor and capital services to raise the sale
value to another capitalist who engages in the next stage of production. The capitalist's decision focuses on their
opportunity cost, the prevailing interest rate which could be realized by
lending the savings to someone else.
Capitalists opt for their own productive activity if the return is
higher than could be realized through lending, and lend out the savings if the
market interest rate exceeds the return to their own production. Thus, if the interest rate falls, less money
will be lent out and more will be used to finance productive activities, and
vice versa.
At the same time, however,
the interest rate influences consumers' decision on how to divide their income between
consumption and saving. The lower the
interest rate, consumers save less and consume more. Any lowering of the interest rate must
simultaneously increase consumption spending, lowering saving, as well as
increase investment in productive activities.
This process would work in reverse when the interest rate rises, if
productive activities could be liquidated rapidly and at low cost.
When the interest rate
rises, capitalists should liquidate their own productive activities to the
extent possible, and lend the money out to take advantage of the higher
return. Ideally, the opposite should
occur when interest rates fall. However,
physical capital comprises illiquid assets, and once savings is invested in
productive activities, it cannot be extracted without delay and loss of
value. Once a capitalist invests in
productive equipment, a higher interest rate may make it desirable to lend out
the money that could be raised by selling the equipment. The sale may involve a delay, however, and as
long as the capitalist enjoys a comparative advantage in the productive
activity, the equipment's selling price must be below what the equipment was
worth to the capitalist.
This cost asymmetry in
converting between financial and physical capital is the basis for Bischoff's
(1970) "putty-clay" model of investment. Uninvested "putty" capital, also
called financial capital, is highly liquid, and can easily be moved from loan
markets into productive activities. Once
savings is tied up in installed physical or "clay" capital, it cannot
be moved costlessly from productive activities back into loan markets, or even
into alternative productive activities.
The Austrian school emphasizes these costs associated with adjusting the
capital structure – also called the structure of production – when interest
rates rise, though it should be kept in mind that similar adjustment costs are
incurred whenever labor, human capital, and raw materials are reallocated. Installed capital equipment can be thought of
as the least adaptable input and the one that most often constitutes a binding
constraint on the process of reallocating production in response to increases
in the interest rate.
In the Austrian view, the
prosperity which precedes a recession is marked by overexpansion of the money
supply above what could be justified by any increase in real consumable output
or productive activities. This could be
seen through a growth of the money supply greater than the growth of real
output, particularly of real consumable output, which unlike investment
spending, is used directly to satisfy individuals' wants. It can also be seen through a lowering of the
nominal interest rate below an appropriate, sustainable, equilibrium interest
rate which would prevail in the absence of monetary overexpansion. While the interest rate is artificially
depressed during the expansion phase, firms invest intensively in physical
capital. Since the interest rate is so
low, the production process can take more time to produce the same amount of real
consumable output, so the production process becomes more drawn out, or more
roundabout, and the slope of the hypotenuse of the Hayekian triangle becomes
flatter, as its base becomes longer.
At the same time, however,
the lower interest rate means consumers save less of their income and consume
more. The below-equilibrium interest
rate results in an economy which takes longer to produce real consumable
output, but also ensures consumers are less willing to wait for their wants to
be satisfied. This production structure
is unsustainable, and must result in abandonment of much capital installed in
early stages of production, and many entrepreneurial plans, as well as high
labor unemployment, even if the interest rate is kept low. Entrepreneurial plans of both producers and
consumers are disrupted because they were predicated on a lower interest rate
and a longer production structure.
In Austrian business cycle theory, the onset of a
recession can occur in any of three ways:
(1) Deflation:
Often, following overexpansion of the money supply, the monetary
authorities will recognize the dangers of the low interest rate and will
intervene to effect adjustment by tightening the money supply. This is signaled by higher interest rates
toward the end of the expansion and the early stages of the recession. This policy response results in a
shorter-lived, though usually more severe, recession, which is the process of
the economy bringing the production process back in line with the sustainable
interest rates and actual time preference.
Contractionary policy can be observed most notably preceding the onset
of the Great Depression, the Volker recession of 1981-82 and the 2001
recession.
(2) Steady inflation: The monetary authorities
continue to inflate the money supply at approximately the same rate as during
the expansion. This would normally occur
whenever the monetary authorities remain unaware of the ill-advised aspects of
their policy-induced credit expansion, or are otherwise innocent of economic
theory. As low interest rates persuade
entrepreneurial managers to invest more in early stages of production and
lower-yielding productive activities, and simultaneously persuade consumers to
save less and consume more, the need for complementary resources required to
simultaneously increase the resource allocation in both early and late stages
of production becomes critical.[1]
Demand for credit finally outstrips the
monetary authority's intended oversupply, driving interest rates up, leading to
massive abandonment of production plans and lower-yielding capital equipment,
precipitating higher unemployment. Most
postwar recessions have started this way, including the first Gulf crisis
recession of 1990-91.
(3) Accelerating inflation: Finally, the monetary authorities may foresee
the recession, or perceive the increased demand for credit, and attempt to
forestall the collapse by increasing the money supply even faster. The economic collapse can be postponed as
long as the monetary expansion proceeds faster than rational individuals can
revise their expectations. This course
of policy is inevitably rendered fruitless as market participants must
eventually learn to anticipate future inflation. This strategy of accelerating inflation may
delay the onset of a recession, but guarantees a more severe and protracted
one. This experience characterized the oil-shock
recessions of 1969-70 and 1973-74.
Productive resources have differing degrees of
substitutability and complementarity (Garrison 1985:168; 2001:49). ABC theory emphasizes the inflexibility
imposed by the high cost of adjusting the production structure by reallocating
installed physical capital. It is
important to realize that similar kinds of inflexibility and high adjustment
costs can come from other resources, particularly labor. Workers often resist seeking employment
outside preferred venues. Because this
source of high unemployment results from high adjustment costs which frustrate
resource allocation and adjustment of the production structure, rather than
from real or nominal wage or price stickiness, this potential cause of
recession, though labor based, should be recognized as Austrian rather than
Keynesian. Mulligan (2002) presents
evidence that labor employment is reallocated over the business cycle in a manner
similar to that predicted by ABC theory for the physical capital it complements. Hayek (1935:136-139) and Garrison (1986:440;
1988; 2001:71-73) draw a fundamental distinction between ordinary changes in
time preference and policy-induced changes in interest rates. Only a decrease in interest rates caused by
credit expansion can drive the business cycle.
According to ABC theory, there should be no cycle if the decrease in interest
rates is due to a general lowering of time preference. Mises (1949:550-566) develops a similar
argument.
3. Qualitative Applications and Earlier Empirics
Austrian business cycle
theory is unmatched in offering persuasive qualitative explanations of historic
business cycles. This fact by itself
makes a powerful case for the Austrian school, which should be accepted as the
dominant macroeconomic policy paradigm. Curiously, the Austrian business cycle
was once the leading theory (Haberler 1937).
More recently the Austrian theory is often dismissed (e.g., Friedman
1969:261-284, 1993; Hummel 1979; Yeager 1986:378; Tullock 1987, 1989; Cowen
1997; Wagner 2000) or simply ignored. In
response, an Austrian literature of defense, apology, and counterattack has
developed (Salerno 1989; Garrison 1996, 2001; Cwik 1998; Block 2001). Although their analysis of investment as a
driver of recession owes little to the Austrian school, Chari, Kehoe, and
McGrattan (2002) conclude the Great Depression was caused by labor market
rigidities, and that investment frictions played a minor role. Holcombe (2001)
discusses some reasons why Austrian macroeconomics is undervalued by the
neoclassical and Keynesian mainstream.
Rothbard's (1963) monumental
study of the inflationary roots of the Great Depression persuasively argues
that credit expansion created an unsustainable boom in the 1920s, and that
government policy frustrated the efforts of economic agents to liquidate
inefficient capital, resulting in a protracted secondary contraction, thus
transforming what would have been a routine recession into the Great Depression
by preventing prompt liquidation of overinvestment. Valuable resources which could have been used
for more productive purposes, and for output more urgently desired by
consumers, instead were tied up in fruitless and counterproductive attempts to
maintain labor employment in the same industries which had already overexpanded
through the malinvestment boom. Focusing
on unorthodox and rarely examined monetary aggregates, Rothbard shows that inflation
and credit expansion continued sporadically well into the 1930s, effectively
preventing any general liquidation of malinvested capital. Rather than facilitate liquidating
malinvestment, easy credit policies generated further opportunities for malinvestment. The misallocation of productive resources was
further exacerbated by governmental efforts to restore and maintain
artificially high prices through cartelization and price controls.
This view contrasts markedly
with Friedman and Schwartz's (1963) conclusion that the secondary contraction
was caused by the Federal Reserve System's failure to provide enough
liquidity (Table 1). Using the standard
monetary aggregate that ultimately emerged as M1, Friedman and Schwartz find
that the main problem during the depression was that the money supply shrank,
even though the monetary base grew.
Table 1 summarizes some of the evidence cited by Keynesian, monetarist,
and Austrian authors. It is difficult to
avoid the conclusion that the Austrian explanation is the most encompassing,
even though Austrian business cycle theory focuses on the unsustainable
expansion which precedes a recession[2]. The monetarists are simultaneously to be
applauded for introducing the first evidence of contractionary policy over
three decades after the start of the recession, as well as to be scolded for
selectively ignoring very real evidence of expansionary policy, which remains
irrefutable.
<<Table 1 about here.>>
The Austrian perspective can
be interpreted as intermediate between the Keynesian, emphasizing a liquidity
trap which made expansionary monetary policy ineffective, and the monetarist,
which criticizes the Fed for unwittingly implementing a contractionary
policy. The Austrian school blames the
expansionary policy of the 1920s for the onset of the Depression, and active
government and central bank policy for transforming what would have been a
routine recession into a decade-long ordeal.
The Austrian school goes beyond the monetarist school in emphasizing the
real discoordination and resource misallocation forced by government and
central bank activism, resulting in persistent and abnormally high
unemployment.
Because he was not an
academic, Harwood (1932) focused only on the unsustainable aspects of
inflation, not on how it created an overextended production structure. Economic historian William Graham Sumner
(1891) also recognized that inflation precipitated economic downturns. Harwood's theory of the business cycle was
that the root cause was any excess of investment spending over saving. Such an imbalance can only be introduced
through systematic expansion of the money supply, which allows banks to lend
funds for business investment in excess of the savings they hold on
deposit. He argued that the amount might
be small initially, but would necessarily grow over time, as producers' goods
face increased demand, bidding up their price.
Harwood agreed with Mises and
Hayek that unsustainable expansion comes about primarily because the interest
rate is kept artificially low due to the oversupply of cheap credit, and
businesses take advantage of the attractive low borrowing rate to finance
expansion of production facilities. He largely disregarded the impact of
localized distortions, recognizing that they occur, but arguing that their
impact distorting the allocation of productive resources must be
negligible. This is a major difference
between Harwood and Mises and Hayek.
In Harwood's view, as soon as
investment spending exceeds saving, businesses that sell producer's goods start
expanding to satisfy increased demand for productive assets. The increased spending results in increased
income to households and workers, meaning that the increased demand is for
consumption goods as well as investment goods.
Harwood's point is that this leads to a general increase in business
activity to satisfy what businesspeople perceive as increased demand for goods
and services. Though he accepted the
Mises and Hayek's views that investment and employment expand fastest and farthest
in the industries most directly affected by the additional investment spending,
he felt this was generally less important than the fact the increase in
spending is quickly diffused throughout the consumption-goods-producing
sector.
O'Driscoll and Shenoy (1976)
present an account of the stagflation of the 1970s. They note that credit expansion increases
nominal demand at the point the newly-created money is injected, distorting the
price vector and the allocation of resources, especially of capital which
cannot be easily reallocated. Credit
expansion always increases consumption expenditures because any new money
results in increased nominal income to some households. Firms engaging in production most remote from
consumption find resource prices bid up, and resources bid away, by firms selling
directly to consumers. Unemployment
starts in these firms remote from final consumption even as prices continue to
be bid up by continued injections of cheap credit. Garrison (2001: 145-164), in the most
important contribution to Austrian macroeconomics since 1949, also provides
convincing accounts of both the Great Depression and the stagflation of the
1970s using the Austrian model.
Cwik (1998) uses the Austrian
theory to analyze the Gulf crisis recession of 1990. Carilli and Dempster (2001) argue that
Austrian business cycle theory places undue reliance on economic agents
misperceiving credit expansion as a real increase in loanable funds. They suggest that even if rational agents
correctly anticipate inflation, agents maximize profits under uncertainty by
taking advantage of the market interest rate whenever it falls below the
underlying rate of time preference.
Keeler (2001) used standardized quarterly data for eight U.S. business
cycles, finding monetary shocks did cause cycles which were propagated through
relative price changes, including nominal interest rates.
Powell's (2002) account of
the Japanese recession of the 1990s is especially noteworthy because he focuses
on exactly how expansionary monetary and fiscal policy recommended to spur
recovery, actually lengthened and deepened Japan's recession. His conclusion is that monetarist policy
prescriptions proved only marginally less ineffective than Keynesian ones. As with the Great Depression, poor policy
prescriptions transformed what should have been a routine recession into a
decade-long ordeal. Mulligan (2002) used
sectoral labor data as indicators of resource allocation among industrial
sectors. Resources are reallocated among
early, middle, and late stages of production in response to changes in nominal
interest rates, as Austrian business cycle theory predicts. Callahan and Garrison (2003) explain the 1990
technology boom and subsequent recession of 2001-2002 in terms of Austrian
business cycle theory. They are able to
point to specific Cantillon effects created when excess liquidity was injected
into localized markets, showing how markets temporarily inflated prices for
computer programmers and web developers, real estate in certain cities, and
technology stocks. Cochrane, Call, and Glahe (2003) argue that the location and
timing of credit injection are especially critical in determining where and how
far the production structure will overexpand, and what will be the nature and
timing of the inevitable collapse.
In marked contrast to
orthodox neoclassical and Keynesian accounts of the business cycle, Austrian
business cycle theory presents a consistent and coherent explanation of the
causes and propagation mechanisms of the business cycle. Though more typically qualitative than
quantitative, the explanatory successes of Austrian business cycle theory have
proved robust over an impressive time period and range of specific
applications. This remarkable success
makes it even more puzzling that Austrian business cycle theory has not been
enthusiastically embraced by non-Austrians, and that it has yet to emerge as
the dominant macroeconomic policy paradigm.
4. Data
This
section documents the data used for econometric estimation and motivates the
choice of data. All data are from the
Federal Reserve Bank of St. Louis Federal Reserve Economic Data (FRED-II)
website.
a. Output Index: The industrial production index (FRED-II
variable INDPRO) is used, reinitialized at January 1959 = 100.
b. Consumption Index: Annualized real personal consumption
expenditures is observed monthly for January 1959 to March 2003 and reported by
the U.S. Department of Commerce Bureau of Economic Analysis (FRED-II variable
PCEC96). This was converted to an index
with January 1959 = 100.
c. Investment Index: An investment index is imputed based on the
difference between total real output and real consumption. Monthly percent growth rates are computed for
the industrial production index and the consumption index. It is assumed that any real output produced
which is not consumer goods is producer goods.
The percent growth rate of the consumption index is subtracted from the
percent growth rate of the industrial production index. The resulting difference is taken as the
imputed percent growth rate for real investment. Starting with January 1959 = 100, the imputed
real investment index for period t + 1 is constructed by multiplying the index
for period t times one plus the imputed percent growth rate.
d. Credit Index: Commercial and industrial loans at all
commercial banks is observed monthly and reported by the Board of Governors of
the Federal Reserve System (FRED-II variable BUSLOANS). This nominal value has to be adjusted for
changes in the price level. The producer
price index (PPI) for all commodities is used as a deflator, which is observed
monthly and reported by the U.S. Department of Labor Bureau of Labor Statistics
(FRED-II variable PPIACO). The deflated
series is converted to an index with January 1959 = 100.
One
difficulty with empirical work which cannot be avoided is that in the Austrian
view, the real value of consumable output is not the objective and observable
exchange value captured in real consumption expenditures, but the subjective
use value extracted by each consumer. This
value is inherently unobservable and disaggregated. Such fundamental issues of methodology and
philosophy help explain why there have been so few econometric analyses of
Austrian theories.
5. The Error-correction Methodology
This
paper proposes the error-correction model as an econometric methodology
especially amenable to interpretation by the Austrian school. Error-correction models provide estimates of
both a structural or equilibrium process toward which adjustment is generally
effected, and the error-correction or disequilibrium adjustment process through
which adjustment is made toward the hypothesized equilibrium. Even if one rejects the reality of any
hypothesized equilibrium, estimates of the disequilibrium adjustment process
still warrant interest.
The
error correction model consists of two parts, a structural equation which
defines the equilibrium process, and a disequilibrium adjustment process. If this equation were estimated by ordinary
least squares or any other econometric technique, the residual of the
structural equation would define the extent of disequilibrium in any given time
period.
The
structural relationships among the four macroeconomic variables will be
normalized with respect to the first three: output, consumption, and
investment. These three variables will
each be expressed in terms of the fourth, commercial and industrial loans. The resulting vector of structural equations
is:
Yt
= a1 + b1Lt + e1t
Ct
= a2 + b2Lt + e2t
It
= a3 + b3Lt + e3t
where a is the intercept,
indicating average output, consumption, and investment in the absence of any
commercial and industrial lending, b is the slope indicating the extent to
which increases in commercial and industrial lending increase output,
consumption, and investment, and e is an additive regression residual or
error. Because the data are
dimensionless constants, the coefficients and residuals are also dimensionless. The vector error correction model is:
DYt = Q1(Yt-1 - a1 - b1Lt-1) + Y1(Ct-1 - a2 - b2Lt-1) + X1(It-1 - a3 - b3Lt-1)
+a11DYt-1 +b11DYt-2 + …+a12DCt-1 +b12DCt-2 + …+a13DIt-1 +b13DIt-2 + …+a14DLt-1 +b14DLt-2 + …+u1t
DCt = Q2(Yt-1 - a1 - b1Lt-1) + Y2(Ct-1 - a2 - b2Lt-1) + X2(It-1 - a3 - b3Lt-1)
+a21DYt-1 +b21DYt-2 + …+a22DCt-1 +b22DCt-2 + …+a23DIt-1 +b23DIt-2 + …+a24DLt-1 +b24DLt-2 + …+u2t
DIt = Q3(Yt-1 - a1 - b1Lt-1) + Y3(Ct-1 - a2 - b2Lt-1) + X3(It-1 - a3 - b3Lt-1)
+a31DYt-1 +b31DYt-2 + …+a32DCt-1 +b32DCt-2 + …+a33DIt-1 +b33DIt-2 + …+a34DLt-1 +b34DLt-2 + …+u3t
DLt = Q4(Yt-1 - a1 - b1Lt-1) + Y4(Ct-1 - a2 - b2Lt-1) + X4(It-1 - a3 - b3Lt-1)
+a41DYt-1 +b41DYt-2 + …+a42DCt-1 +b42DCt-2 + …+a43DIt-1 +b43DIt-2 + …+a44DLt-1 +b44DLt-2 + …+u4t
Note
the expressions in parentheses are lagged residuals from the structural
equations, and thus could be represented simply by (et-1)s. These are the errors which the
disequilibrium adjustment process of the error correction model attempts to
explain. The upper-case Greek letters
are the structural adjustment or disequilibrium adjustment terms, which weight
the error-correction processes and so indicate the importance of the past
changes in the explanatory variables in effecting adjustment toward the
hypothesized equilibrium. The equilibrium
represented by the structural equations is generally never realized, and if
realized, is not persistent. If
equilibrium is ever reached, that is represented by zero residuals in the
structural equations for those observations.
Whenever residuals are non-zero, that is, whenever the system is in
disequilibrium, which generally will be for virtually every observation, the
non-zero residual in period t results in an adjustment back toward equilibrium
in period t+1, represented by the error-correction processes. The error-correction processes can be thought
of as indicating how the data processes can best be represented as adjusting to
maintain the long-run equilibrium.
Conventional
inference is valid in an error-correction model even when the structural
variables are nonstationary, provided the residuals are white-noise processes
with no serial correlation. It is
generally assumed that adding a sufficient number of lagged difference terms in
the disequilibrium adjustment process is always sufficient to guarantee
white-noise errors.
6. The Vector Error-correction Model
This
section presents and interprets empirical estimates based on a simple parameterization
of Austrian business cycle theory. In
the subjectivist theory of a capital-using economy, entrepreneurial planners
act as the subjects of productive activities, creating real consumable output
as the object (Garrison 1985:164-165; 2001:15). Interest rates facilitate
intertemporal coordination of productive resources by clearing the loanable
funds market (Garrison 1986:440; 2001:39).
In this regard disequilibrium interest rates play the same role as
prices in signaling opportunities for entrepreneurial discovery (Kirzner
1984a:146; 1984b:160-161; 1997), and individual entrepreneurs respond by
maintaining the production structure, that is, they adjust it by reallocating
resources.
a. Unit Root and Cointegration Tests
Most
macroeconomic time series display an increasing trend, and unit root tests were
developed to identify this characteristic.
Stationary time series are said to have zero roots or be integrated of
order zero [I(0)]. Non-stationary series
may have a unit root or be first-order integrated [I(1)]. Unit root series become I(0) when
first-differenced. Regressions estimated
with non-stationary data will not have the white-noise residuals needed for
valid inference. The regression could be
estimated in first-differences, but then any long-term information carried by
the levels of the variables is lost.
Error-correction models overcome this difficulty by estimating a
regression in first-differences augmented by error-correction terms, the lagged
differences between the actual and estimated value of the left-hand-side
variable, collectively referred to as the error-correction process, also called
the disequilibrium adjustment process.
The coefficients on the first-differenced variables constitute the
cointegrating vector or structural relationship. A sufficient number of lagged
error-correction terms are added to guarantee white-noise errors and valid
inference (Davidson and McKinnon 1993:720-730, Kennedy 1998:266-270).
The
Johansen-Juselius (1990) procedure was used to identify stable, long-term
relationships between real consumable output and the interest rate term
spread. Table 2 reports augmented
Dickey-Fuller (1979) and Phillips-Perron (1988) unit-root tests for each variable. The augmented Dickey-Fuller results with 48
lags indicate output, investment, and commercial and industrial loans are all I(1),
but that consumption may be I(2) or integrated of higher order. Phillips-Perron tests indicate all variables
are I(1).
<<Table 2
about here.>>
Table
3 reports Johansen-Juselius tests for cointegration. Results of the trace test, a likelihood
ratio, indicate a stable, cointegrated relationship among the system of four
macroeconomic variables with three cointegrating vectors.
<<Table 3
about here.>>
Because
the four variables in the model are cointegrated, ordinary least squares
estimates of the structural relationships have the property of
superconsistency.
b. The Cointegration Space
The
estimate of the vector error correction model (VECM) is reported in table 4. To facilitate interpretation, the VECM is
normalized with respect to and solved for output, consumption, and
investment. Coefficients on the forty-eight
lagged difference terms are not reported, partly due to space limitations, and
also because individual coefficient estimates hold limited interest. The implications of the disequilibrium
adjustment process can be inferred from the variance decomposition and impulse
response graphs (Figures 2-4).
<<Table 4
about here.>>
The
slope coefficient in the structural equation for the industrial production
index is not significant, indicating that credit expansion as measured by
commercial and industrial loans, does not significantly affect real output
either positively or negatively. The
slope coefficient in the structural equation for consumption is positive and
significant, indicating that credit expansion leads to higher consumption
expenditures. This result is consistent
with Austrian business cycle theory, which suggests consumers save less in
response to the lower interest rate. The
slope coefficient in the structural equation for investment is negative and
significant, suggesting that credit expansion lowers investment expenditure.
Austrian business cycle theory suggests that credit expansion shifts
resource allocation away from middle stages of production toward early and late
stages (consumption).
Adjusted
R-square for the disequilibrium adjustment processes are very low. In spite of the low R-squares, both disequilibrium adjustment terms [Q, Y, and X] are positive and
significant, only in the disequilibrium adjustment process for
consumption. This is an especially
interesting result, which is easy to account for according to Austrian business
cycle theory. Apparently any market
disequilibria, measured by non-zero residuals in any of the three structural
equations, effects correction chiefly through changes in consumption
spending. Little or no adjustment occurs
through total output or through investment.
Consumer behavior is highly responsive to market disequilibria, but
producer behavior exhibits much more inertia, likely due to the fixed capital
embodied in the production structure.
The
specification of the disequilibrium adjustment process includes only lagged
first-differences of output, consumption, investment, and commercial and
industrial loans, one through forty-eight, four years of lagged differences. The median length of a recession is somewhat
less than two years, but the median length of an expansion may be as long as
ten years. One approach would be to
average the two figures to ensure capturing most of the dynamics in the
disequilibrium adjustment process. However,
the average expansion generally cannot last so long if it is characterized by
policy-induced credit expansion. The
complete dynamics of the business cycle may be captured with four to five years
of lagged differences. This would be the
case if a recession always results after so many years of credit expansion, and
if the recessions are always shorter than the expansions.
Validity
of the error-correction specification depends on cointegration among the
variables in the model and white-noise characteristics of the residuals. Jarque-Bera (1980) tests of normality of the
residuals are reported in Table 5. Results
strongly suggest the residual series are not multivariate normal. It should be emphasized, however, that
normality is a sufficient, rather than a necessary, condition for valid VECM
estimates. The Johansen-Juselius procedure
estimates the VECM by maximum likelihood, imposing the most nearly normal
character possible on the residuals.
<<Table 5 about
here.>>
Non-normal
residuals can be interpreted as evidence of specification error, and from the
perspective of the Austrian school, specification error is necessarily present
in all econometric models. The measures
of real output, real consumable output, and real investment are not the
subjective use value extracted by users, and the real interest rate measure is
not the idealized interest rate hypothesized by Hayek and Mises (Garrison
1985:169-170; 2001:50; Rothbard 1970:321-323).
Thus, Austrian methodological arguments suggest an a priori expectation
of unavoidable misspecification and measurement error in any econometric
empirical work.
c. Granger Causality Tests
Granger causality tests (Granger 1969)
are presented in table 6, indicating rejection of the null hypotheses that the forty-eight
lagged differences of the four macroeconomic indices can be deleted from the disequilibrium
adjustment processes for output, investment, or commercial and industrial loans,
but failing to reject the hypothesis that the lagged differences can be deleted
from the disequilibrium adjustment process for consumption. Thus consumption is endogenous with respect
to the remaining three exogenous variables.
This test is strongly dependent on the VECM estimate and the maintained
hypothesis that all relevant variables have been included in the VECM (Davidson
and MacKinnon 1993:686).
Although ABC theory asserts that real consumable
output depends on the stability of the money supply and the maintenance of an
appropriate, sustainable interest rate, it might reasonably be questioned
whether all relevant variables have been included, especially in light of the
Austrian school’s methodological criticisms of output and interest rate
measures. Orthodox neoclassical and
Keynesian economists could contribute additional reasons to suspect omitted
variables. The Granger causality tests
should be viewed as inherently context dependent.
This outcome supports the interpretation
that most adjustment to disequilibrium occurs through consumption, rather than
investment, due to the high costs of adjusting the production structure
characterized by multispecific capital.
The lagged difference terms play little role in adjusting consumption
spending toward equilibrium.
<<Table 6 about here.>>
d. Impulse Response Functions
Graphs
of the impulse response functions are presented in figures 2 and 3. In each figure, the right-hand graphs in each
row are the ones of interest for Austrian business cycle theory. It indicates that over the period studied, a
one standard-deviation increase in real commercial and industrial lending has
resulted, on average, in a two standard-deviation decrease in total industrial
production, a one standard-deviation decrease in real consumption, and
approximately a 0.6 standard-deviation decrease in investment, after four
years. Impulse response functions measure
the strength of the disequilibrium adjustment processes working through each
variable. The fact that disequilibrium
adjustment is effected downward on output, consumption, and investment,
whenever there is a positive shock to commercial and industrial loans, is
strong support for Austrian business cycle theory.
<<Figure 2 about
here.>>
Figure 3 shows cumulative
impulse response functions. Again,
focusing on the right-hand graphs in each row, these illustrate that increases
in commercial and industrial loans, force a large downward adjustment on output
(40 standard deviations after four years), consumption (25 standard deviations
after four years), and investment (12 standard deviations after four
years). The interpretation suggested by
Austrian business cycle theory is that credit expansion, manifested by
exogenous shocks to commercial and industrial loans, causes scarce capital
resources to be misallocated over an unsustainably long and low-yielding
production structure. Too much capital
is allocated to early and late stages, with too little allocated to the
critical middle stages which are necessary to transform early stage
goods-in-process into late-stage consumable output. This culture of waste and misallocation
permanently shifts the economy into a lower growth trajectory.
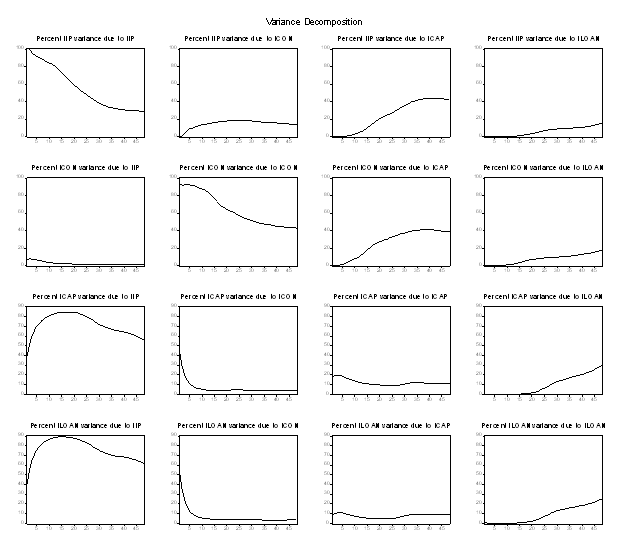
e. Variance Decomposition Functions
Graphs
of the variance decomposition functions are presented in figure 4. Again, the right-hand graph in each row is
the one of interest for Austrian business cycle theory. These graphs indicates that after four years
or 48 months, approximately 20% of the variance in industrial production,
consumption, investment, and commercial and industrial loans has been
attributable to variation in business and commercial loans, over the period
studied. Interestingly, while
significant variation seems to transfer from industrial production to
commercial and industrial loans, very little variation seems to be transmitted
from consumption or investment to commercial and industrial loans.
<<Figure 3 about
here.>>
7. Conclusion
This
paper presents evidence of cointegration among real output, consumption,
investment, and commercial and industrial loans. This finding implies a close, stable relationship
among these four macroeconomic variables.
Austrian business cycle theory is applied to interpret these empirical
regularities. A simple vector
error-correction model is specified and presented, and demonstrated to have a
great deal of explanatory power over 1959-2003 historical data.
Cointegration
analysis identifies a stable long-term relationship or cointegrating vector,
which constitutes a dynamic equilibrium entrepreneurial planners have generally
effected adjustment toward during the 1959-2003 observation period. This equilibrium is not necessarily ever
realized. The market process consists of
entrepreneurial planners effecting adjustment toward a dynamic equilibrium they
continuously redefine. The prevailing
term structure of interest rates determines resource allocation among early,
middle, or late stages of production, allocating resources and production in
accordance with consumers' time preference and available investment
alternatives. Estimates of a stable
long-run relationship using
ABC
theory is founded on the concept of a sustainable, market-determined interest
rate, and predicts negative consequences when that equilibrium is persistently
disturbed. Economists and laypeople are
well aware of these consequences: the periodic high unemployment associated
with the business cycle. The policy
prescriptions of the Austrian school are unmistakable: first, never disturb the
interest rate with credit expansion or monetary inflation, and second, after
the first policy prescription has been violated, never interfere with
entrepreneurial planners' efforts to liquidate suboptimal production plans as
rapidly as possible. As long as
economists and policy makers believe the business cycle can be avoided through
the activism of charismatic central bankers, recessions will be inevitable.
References
Bischoff, Charles W. (1970).
"A Model of Nonresidential Construction in the
Breusch, T.S., and Godfrey,
L.G. (1986). "Data Transformation Tests," Economic Journal 96: 47-58.
Callahan, Gene; and Garrison, Roger W. (2003). Does
Austrian Business Cycle Theory Help Explain the Dot-com Boom and Bust? Quarterly Journal of Austrian Economics
6(2): 67-98.
Carilli, Anthony M. and
Dempster, Gregory M. (2001). "Expectations in Austrian Business Cycle
Theory: an Application of the Prisoner's Dilemma," Review of Austrian
Economics 14(4): 319-330.
Cowen, Tyler (1997). Risk and Business Cycles. London: Routledge.
Davidson, Russell, and
McKinnon, James G. (1993). Estimation and
Inference in Econometrics,
Dickey, D.A., and Fuller,
W.A. (1979). "Distribution of the Estimators for Autoregressive Time
Series with a Unit Root," Journal of the American Statistical
Association 74: 427-431.
Federal Reserve Bank of
Friedman, Milton and Schwartz, Anna J. (1963). A Monetary History of
the
Garrison, Roger W. (1978).
"Austrian Macroeconomics: a Diagrammatical Exposition," in Spadaro,
Louis M., ed., New Directions in Austrian
Economics,
Garrison, Roger W.
(1985)."A Subjectivist Theory of a Capital-Using Economy," in
O’Driscoll, G.P. and Rizzo, M., The
Economics of Time and Ignorance,
Garrison, Roger W. (1986).
"The Hayekian Trade Cycle Theory: a Reappraisal," Cato Journal 6(2): 437-453.
Garrison, Roger W. (1988).
"The Austrian Theory of the Business Cycle in Light of Modern
Macroeconomics," Review of Austrian
Economics 3: 3-29.
Garrison, Roger W. (1995).
"Equilibrium and Entrepreneurship," Advances in Austrian Economics 2: 67-78.
Garrison, Roger W. (2001). Time and Money: the Macroeconomics of
Capital Structure,
Granger, C.W.J. (1969). "Investigating Causal Relationships by
Econometric Models and Cross-spectral Methods," Econometrica 37: 424-438.
Haberler, Gottfried. ([1937] 1960). Prosperity and Depression: a
Theoretical Analysis of Cyclical Movements (2nd ed.)
Harwood, E.C. ([1932] 1974). Cause and Control of the Business Cycle
(10th ed.) Great
Hayek, F.A. (1931). Prices and Production (1st ed.)
Hayek, F.A. ([1933] 1966). Monetary Theory and the Trade Cycle,
Hayek, F.A. ([1935] 1967). Prices and Production (2nd ed.)
Hayek, F.A. ([1939] 1969). Profits, Interest, and Investment, and Other
Essays on the Theory of Industrial Fluctuations, reprint,
Hayek, F.A. (1941). The Pure Theory of Capital,
Hicks, J.R. ([1939] 1946). Value and Capital: an Inquiry into Some
Fundamental Principles of Economic Theory, 2nd ed.,
Hülsmann,
Jörg Guido (2001). Garrisonian Macroeconomics. Quarterly Journal of Austrian Economics 4(3): 33-42.
Hummel,
Jeffrey Rogers (1979). Problems with Austrian Business Cycle Theory. Reason Papers 5: 41-53.
Jarque, C.M., and A.K. Bera
(1980). "Efficient Tests for Normality, Homoskedasticity, and Serial
Johansen, Søren (1995). Likelihood-based Inference in Cointegrated
Vector Auto-regressive Models,
Johansen, Søren, and
Juselius, Katerina (1990). "Maximum Likelihood Estimation and Inference on
Cointegration – with Applications to the Demand for Money,"
Keeler, James P. (2001). "Empirical Evidence on the Austrian Business Cycle Theory," Review of Austrian Economics 14(4): 331-351.
Kennedy, Peter (1998). A Guide to Econometrics (4th ed.)
Keynes, John Maynard (1936).
The General Theory of Employment,
Interest, and Money,
Lachmann, Ludwig M. (1956). Capital and Its Structure,
Lombardo, Gary A.; and Mulligan, Robert F. (2003).
Resource Allocation: A Hayekian Paradigm for Maritime Conglomerates. Quarterly Journal of Austrian Economics 6(1):
3-21.
Lütekpohl, Helmut. (1991). Introduction
to Multiple Time Series Analysis,
Miller, Arthur (1968). The Price. New York: Viking.
Mises, Ludwig von. ([1912] 1980).
The Theory of Money and Credit,
Mises, Ludwig von. ([1949]
1998). Human Action (5th ed.)
Modigliani, Franco (1944).
"Liquidity Preference in the Theory of Interest and Money," Econometrica 12: 45-88, reprinted in
Hazlitt, Henry, ed., The Critics of
Keynesian Economics,
Mueller, Antony P. (2001). Financial Cycles, Business
Activity, and the Stock Market. Quarterly
Journal of Austrian Economics 4(1): 3-22.
Mulligan, Robert F. (2002). A Hayekian Analysis of the
Term Structure of Production. Quarterly Journal of Austrian Economics
5(2): 17-33.
Mulligan, Robert F. (2003). An Empirical Examination
of Austrian Business Cycle Theory.
Western Carolina University, Department of Business Computer Information
Systems and Economics.
Mulligan, Robert F. (2004). Accounting for the
Business Cycle: Nominal Rigidities, Factor Heterogeneity, and Austrian Capital
Theory. Western Carolina University,
Department of Business Computer Information Systems and Economics.
Newley, W. and West, K.
(1987). "A Simple Positive Semi-definite, Heteroskedasticity and
Autocorrelation Consistent Covariance Matrix," Econometrica 55:
703-708.
O'Driscoll, Gerald P. and
Rizzo, Mario. (1995). The Economics of
Time and Ignorance,
O'Driscoll, Gerald P. and Shenoy, Sudha R. (1976). "Inflation,
Recession, and Stagflation," in Dolan, Edwin G., ed., The
Foundations of Modern Austrian Economics,
Osterwald-Lenum, Maurice. (1992). "A Note with Quantiles of the
Asymptotic Distribution of the Maximum Likelihood Cointegration Rank Test
Statistics,"
Phillips, P.C.B. and Perron, P. (1988). "Testing for a Unit Root
in Time Series Regression," Biometrika
75: 335-346.
Powell, Benjamin (2002). "Explaining
Rothbard, Murray N. ([1962]
2000).
Rothbard, Murray N. ([1963]
2000). Man, Economy, and State,
Salerno, Joseph T. (1989).
Comment on Tullock's "Why Austrians are Wrong about Depressions." Review of Austrian Economics 3:141-145.
Salerno, Joseph T. (2001).
Does the Concept of Secular Growth Have a Place in Capital-based
Macroeconomics? Quarterly Journal of Austrian Economics 4(3): 43-62.
Sumner, William Graham.
(1891). The Financier and the Finances of
the American Revolution, 2 vols., New York: Dodd and Mead.
Tullock, Gordon (1987). Why the Austrians are Wrong
about Depressions. Review of Austrian
Economics 2: 73-78.
Tullock, Gordon (1989). Reply to Comment by Joseph T.
Salerno. Review of Austrian Economics
3: 147-149.
Wagner, Richard E. (1999). Austrian Cycle Theory:
Saving the Wheat while Discarding the Chaff. Review of Austrian Economics 12(1): 65-80.
Yeager, Leland (1986). The Significance of Monetary
Disequilibrium. Cato Journal
6:369-399.
|
Table 1 Competing Views of the Great Depression |
||
|
Keynesian |
Monetarist |
Austrian |
|
Liquidity
trap created once nominal interest rates became low enough; bank demand for
excess reserves became perfectly elastic.
Monetary base doubled between 1929-38: monetary policy was
expansionary, but excess reserves accumulated in banks. Demand for loans depressed due to
unfavorable business outlook. Banks
did not buy short-term securities because nominal yields were so low. |
Real
interest rates extremely high due to price deflation: e.g., CPI fell 10% in
1931 and 1932. Indicates
contractionary policy. Growth in
monetary base mostly attributable to currency held by public, unavailable to
be loaned out, rather than bank reserves.
"Flight to quality" greatly increased demand for short-term
Treasury securities, depressing their yield.
Fed tightened discount lending policy in 1931, and doubled reserve
requirement between 1936-37, triggering a secondary recession. |
Expansionary
monetary policy depressed interest rates and created unsustainable investment
boom throughout late 1920s. Monetary
policy was intermittently both expansionary and contractionary throughout the
1930s. Government intervention
initiated under the Hoover administration between 1930-32 delayed liquidation
of malinvested capital. Price fixing,
fiscal stimulus, and inconsistent monetary activism, continued and extended
under the Roosevelt administration, prevented liquidation of malinvested
capital, prolonging the contraction. |
|
Keynes 1936, Hicks
1939, Modigliani 1944 |
Friedman and
Schwartz 1963: 411-419 |
Rothbard 1962,
Garrison 2001 |
|
Table 2 Unit Root Tests January 1959 – December 2003 |
||||
|
Augmented Dickey-Fuller Tests 48 lags |
||||
|
Variable |
Levels |
First
differences |
||
|
Intercept |
Intercept
+ trend |
Intercept |
Intercept
+ trend |
|
|
Industrial
Prod Index |
0.7050 |
-1.1001 |
*** -3.5254 |
** -3.6559 |
|
Consumption
Index |
2.6358 |
1.3121 |
-1.3826 |
-2.5878 |
|
Investment
Index |
-0.3907 |
-2.9062 |
*** -3.5876 |
** -3.6919 |
|
Credit
Index |
-0.4277 |
-2.9557 |
** -3.2375 |
* -3.3631 |
|
Critical values2 |
1% -3.4459 5% -2.8677 10% -2.5700 |
1% -3.9810 5% -3.4209 10% -3.1329 |
1% -3.4460 5% -2.8677 10% -2.5701 |
1% -3.9796 5% -3.4202 10% -3.1325 |
|
Phillips-Perron
Tests 5 lag
truncation for Bartlett kernel (Newley and West 1987) |
||||
|
Industrial
Prod Index |
0.5148 |
-1.4103 |
*** -16.9202 |
*** -16.9411 |
|
Consumption
Index |
6.1908 |
1.5984 |
*** -27.8791 |
*** -29.8532 |
|
Investment
Index |
-0.8417 |
-2.6552 |
*** -19.6074 |
*** -19.6153 |
|
Credit
Index |
-0.6497 |
-2.4878 |
*** -19.8052 |
*** -19.8242 |
|
Critical values |
1% -3.4448 5% -2.8672 10% -2.5698 |
1% -3.9795 5% -3.4202 10% -3.1324 |
1% -3.4448 5% -2.8672 10% -2.5698 |
1% -3.9795 5% -3.4202 10% -3.1324 |
|
Notes:
|
||||
|
Table 3 Tests for Cointegration among Indexes of
Industrial Production, Consumption, Investment, and Commerical and Industrial
Loans December 1959 – December 2003 (491 observations after adjusting endpoints
with 48 lag intervals) |
||||
|
Hypothesized # CE(s) |
Maximum Eigenvalue |
Trace Statistic |
5% Critical Value |
1% Critical Value |
|
None ** |
0.087577 |
85.59848 |
53.12 |
60.16 |
|
At most 1 * |
0.035336 |
40.59756 |
34.91 |
41.07 |
|
At most 2 * |
0.031952 |
22.93381 |
19.96 |
24.60 |
|
At most 3 |
0.014134 |
6.989258 |
9.24 |
12.97 |
|
Notes:
|
||||
|
Table 4 Vector Error
Correction Model Industrial
Production, Consumption, and Investment explained by Commercial and Industrial Loans December 1959 – December
2003 (491 observations after adjusting endpoints) |
|||||||
Cointegrating
equations
|
|||||||
|
|
Industrial
Production Index
|
Consumption
Index
|
Investment Index |
||||
Constant
|
248.4555 |
459.6424 |
-200.7269 |
||||
|
(174.647) |
(190.536) |
(2.24949) |
|||||
|
[ 1.42262] |
[ 2.41236] |
[-89.2322] |
|||||
Index
of Commercial & Industrial Loans
|
-3.407440 |
-5.402801 |
1.015573 |
||||
|
(1.88005) |
(2.05110) |
(0.02422) |
|||||
|
[-1.81242] |
[-2.63410] |
[ 41.9390] |
|||||
Error
correction process
Summary
Statistics |
|||||||
|
|
D(IIP) |
D(Cons) |
D(Invest) |
D(C & I Loans) |
|||
Cointegrating Equation 1 coefficients
|
-0.148849 |
-0.377618 |
0.036580 |
-0.063900 |
|||
|
(0.08697) |
(0.08391) |
(0.04271) |
(0.04339) |
||||
|
[-1.71141] |
[-4.50008] |
[ 0.85652] |
[-1.47281] |
||||
|
Cointegrating Equation 2
coefficients |
0.136683 |
0.360550 |
-0.037930 |
0.063729 |
|||
|
(0.08214) |
(0.07925) |
(0.04033) |
(0.04097) |
||||
|
[ 1.66411] |
[ 4.54981] |
[-0.94046] |
[ 1.55538] |
||||
|
Cointegrating Equation 3
coefficients |
-0.638940 |
-2.808482 |
0.389831 |
-0.622957 |
|||
|
(0.66308) |
(0.63974) |
(0.32559) |
(0.33077) |
||||
|
[-0.96360] |
[-4.39003] |
[ 1.19730] |
[-1.88334] |
||||
|
R-square |
0.554330 |
0.454815 |
0.501887 |
0.506806 |
|||
|
Adjusted R-square |
0.262236 |
0.097498 |
0.175421 |
0.183564 |
|||
|
Sum of squared residuals |
627.0531 |
583.6946 |
151.1913 |
156.0405 |
|||
|
Standard error of equation |
1.455480 |
1.404258 |
0.714690 |
0.726061 |
|||
|
F-statistic (zero slopes) |
1.897779 |
1.272863 |
1.537332 |
1.567884 |
|||
|
Logarithm of likelihood function |
-756.7449 |
-739.1541 |
-407.5248 |
-415.2752 |
|||
|
Akaike information criteria AIC |
3.876761 |
3.805108 |
2.454276 |
2.485846 |
|||
|
Schwarz criteria SC |
5.543374 |
5.471720 |
4.120888 |
4.152458 |
|||
|
Mean of dependent variables |
0.599631 |
0.775520 |
-0.039485 |
0.044803 |
|||
|
Standard deviation of dependent variables |
1.694523 |
1.478165 |
0.787048 |
0.803548 |
|||
|
Determinant of residual covariance matrix |
0.001614 |
||||||
|
Logarithm of likelihood function |
-711.5076 |
||||||
|
Logarithm of likelihood function adjusted
for degrees of freedom |
-1208.483 |
||||||
|
Akaike information criterion AIC |
8.160826 |
||||||
|
Schwarz criterion |
14.95548 |
||||||
|
Notes:
|
|||||||
|
Table 5 Vector Error Correction Model Jarque-Bera Test for Multivariate Normality
of Residuals |
||||
|
Component |
Skewness |
Chi-square |
d.f. |
Probability
|
|
IIP |
-0.020213 |
0.033433 |
1 |
0.8549 |
|
Consumption Index |
0.073538 |
0.442536 |
1 |
0.5059 |
|
Investment Index |
-0.062424 |
0.318888 |
1 |
0.5723 |
|
C&I Loans
Index |
0.023723 |
0.046056 |
1 |
0.8301 |
|
Joint Test |
|
0.840912 |
4 |
0.9329 |
|
|
Kurtosis |
Chi-square |
d.f. |
Probability
|
|
IIP |
1.360762 |
54.97361 |
1 |
0.0000 |
|
Consumption Index |
1.439926 |
49.79210 |
1 |
0.0000 |
|
Investment Index |
1.591071 |
40.61143 |
1 |
0.0000 |
|
C&I Loans
Index |
1.563641 |
42.20816 |
1 |
0.0000 |
|
Joint Test |
|
187.5853 |
4 |
0.0000 |
|
|
|
Jarque-Bera |
d.f. |
Probability
|
|
IIP |
|
55.00705 |
2 |
0.0000 |
|
Consumption Index |
|
50.23464 |
2 |
0.0000 |
|
Investment Index |
|
40.93031 |
2 |
0.0000 |
|
C&I Loans
Index |
|
42.25421 |
2 |
0.0000 |
|
Joint Test |
|
188.4262 |
8 |
0.0000 |
|
Notes:
3.
Cholesky
orhogonalization (Lütkepohl 1991). |
||||
|
Table 6 Wald Tests for VECM Pairwise Granger Causality/Block Exogeneity |
|||
|
Dependent
variable: D(IIP) |
|||
|
Exclude |
Chi-sq |
df |
Prob. |
|
D(ICON) |
56.80302 |
48 |
0.1799 |
|
D(ICAP) |
51.27748 |
48 |
0.3465 |
|
D(ILOAN) |
52.69083 |
48 |
0.2975 |
|
All |
186.5244 |
144 |
0.0098 |
|
Dependent
variable: D(ICON) |
|||
|
Exclude |
Chi-sq |
df |
Prob. |
|
D(IIP) |
62.32825 |
48 |
0.0801 |
|
D(ICAP) |
41.75657 |
48 |
0.7252 |
|
D(ILOAN) |
49.76012 |
48 |
0.4031 |
|
All |
131.1024 |
144 |
0.7717 |
|
Dependent
variable: D(ICAP) |
|||
|
Exclude |
Chi-sq |
df |
Prob. |
|
D(IIP) |
50.22959 |
48 |
0.3852 |
|
D(ICON) |
55.01674 |
48 |
0.2262 |
|
D(ILOAN) |
49.14254 |
48 |
0.4271 |
|
All |
210.5146 |
144 |
0.0003 |
|
Dependent
variable: D(ILOAN) |
|||
|
Exclude |
Chi-sq |
df |
Prob. |
|
D(IIP) |
46.63902 |
48 |
0.5287 |
|
D(ICON) |
55.77311 |
48 |
0.2057 |
|
D(ICAP) |
45.06220 |
48 |
0.5940 |
|
All |
230.8588 |
144 |
0.0000 |
|
Notes:
Granger
causality tests are sensitive to changes in model specification, including
but not limited to, lag structure, intercepts, and variables included
(Davidson and MacKinnon 1993: 629-31). |
|||
Figure 11
The Hayekian
Triangle: Production and Capital Structure
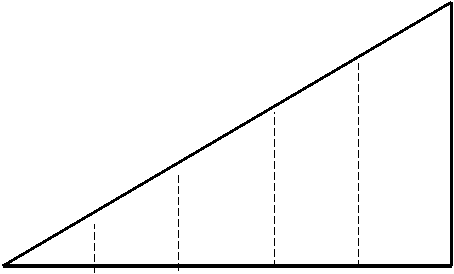

![]()

Production Time
1 Garrison
2001, p. 47.
Figure 2
Vector Error Correction Model
Impulse Response Functions
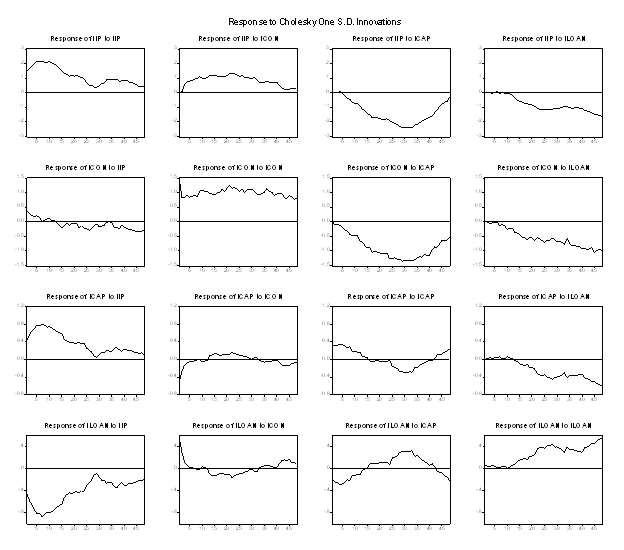
Figure 3
Vector Error Correction Model
Cumulative Impulse Response

Figure 4
Vector Error Correction Model
Variance Decomposition